Politico has a detailed investigation of the “Young Republicans” in America, based on leaked chat messages like these.
Current reporting of a secret Republican platform (e.g. “invisible empire”) sounds very familiar to me, as a disinformation historian.
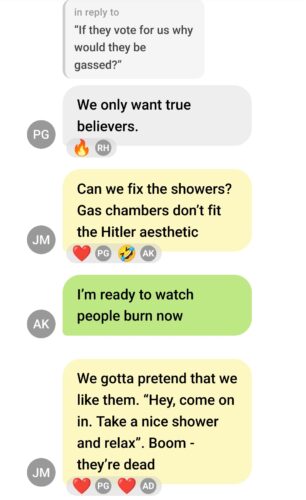
They referred to Black people as monkeys and “the watermelon people” and mused about putting their political opponents in gas chambers. They talked about raping their enemies and driving them to suicide and lauded Republicans who they believed support slavery.
How familiar? I present the canonical Ronald Reagan example.

The Young Republicans knew exactly what they were doing:
“If we ever had a leak of this chat we would be cooked fr fr” heart emoji
They understood the content was genuinely extreme, not actually funny.
The “joke” framing was explicitly strategic cover using information warfare tactics, camouflage.
The dangerous tell:
When something appears over 251 times in chats, with specific known violent hate symbols (1488), and includes detailed policy discussions interwoven with the “jokes,” it stops being humor and becomes domestic terrorist ideology with a thin comedic veneer.
The jester’s protection only works when everyone knows it’s performance. What happens when the jester starts believing their own act, and then acting on their beliefs?
Giunta wrote “everyone that votes no is going to the gas chamber” while actually running a political campaign. That means he made an articulated threat wrapped in historical genocide imagery, for use as an organizing tool.
The Vice President “disciple” of the ACTS 17 (Nazism) extremist preacher Peter Thiel who put him into power – JD Vance – has waded into the topic by deflecting from and minimizing Republican love of Hitler.
When the second-highest elected official provides cover for “I love Hitler” and gas chamber jokes as mere “college” banter, the performance has become policy position. This trajectory has obvious historical precedents.
The hypocrisy about Nazism is stark:
Vance spent years characterizing campus speech as dangerous indoctrination requiring “aggressive attack,” even titling a 2021 speech “The Universities Are the Enemy.”
Vance specifically characterized universities as dangerous places that “indoctrinate students” with “political orthodoxy” and said they peddle “deceit and lies.” Vance quoted Richard Nixon’s line “The professors are the enemy” approvingly, stating there was “wisdom” in Nixon’s words.
Yet when Young Republican leaders – including government officials and state legislators – express explicit support for Hitler and make threats about gas chambers, Vance dismisses it as just… wait for it… normal “college group chat”.
Apparently to the Vice President, discussing any opposition to Nazism on campus constitutes dangerous indoctrination worthy of “aggressive attack,” while expressing extreme support for Hitler and threatening political opponents with execution by gas chamber is harmless college “humor.”
What’s the historical outcome when leadership actively protects, rather than condemns, organized imminent extremist violence in discourse?
- Weimar Germany (1920s-30s): Leadership initially dismissed Nazi rhetoric as fringe or protected it as “free speech”, enabling genocide
- Rwanda (early 1990s): Radio stations normalized dehumanizing language; political leadership either participated or stayed silent, enabling genocide
- Former Yugoslavia (1980s-90s): Nationalist leaders used historical grievances and ethnic dehumanization in “joking” contexts before committing genocide
American courts have long recognized that speech itself can constitute actionable harm when it incites imminent lawless action (Brandenburg v. Ohio), communicates true threats (Virginia v. Black), or inflicts injury by its very utterance (Chaplinsky v. New Hampshire).
The Young Republicans’ messages are explicit threats against specific political targets, combined with organizing capacity and historical genocide imagery.
They very clearly meet the legal thresholds.